Find Equation of Line From 2 Points

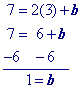
There are a few different ways to write the equation of line .

Slope Intercept Form

The first half of this page will focus on writing the equation in slope intercept form like example 1 below.

Point Slope Form

However, if you are comfortable using the point slope form of a line, then skip to the second part of this page because writing the equation from 2 points is easier with point slope form.

Which Form is better?

Point Slope Form is better

Point slope form requires fewer steps and fewer calculations overall. This page will explore both approaches. You can click here to see a side by side comparison of the 2 forms.

Video Tutorial
on Finding the Equation of a line From 2 points

Example - Slope Intercept Form

Using Slope Intercept Form

Find the equation of a line through the points (3, 7) and (5, 11)

$$ y = \red x + b \\ y = \red 2 x + b $$

Substitute either point into the equation. You can use either $$(3, 7)$$ or $$(5, 11)$$ .

Let's use $$( \red 3, \red 7)$$

$$ y = 2x + b \\ \red 7 = 2 (\red 3) + b $$

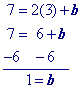
Step 4 the y intercept

Substitute $$ 1$$ for $$ \red b $$ , into the equation from step 2.

$$ y = 2x + \red b \\ y = 2x + \red 1 \\ \boxed < y = 2x + 1 >$$

Use our Calculator

You can use the calculator below to find the equation of a line from any two points. Just type numbers into the boxes below and the calculator (which has its own page here) will automatically calculate the equation of line in point slope and slope intercept forms.

Toggle Points

(This link will show the same work that you can see on this page)

Practice Problems- Slope Intercept Form

Problem 1

Find the equation of a line through the following 2 points: (4, 5) and (8, 7)

step 1 find slope

Substitute either point into the equation. You can use either (4, 5) or (8, 7) .

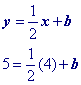
Step 3

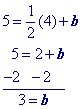
Step 4 the y intercept

Substitute b, 3, into the equation from step 2.

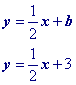
Final Equation of Line

Problem 2

Find the equation of a line through the following the points: (-6, 7) and (-9, 8).

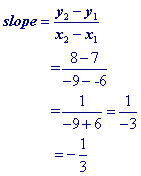
step 1 find slope

Substitute the slope for 'm' in the slope intercept equation.

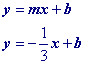
equation of line

Substitute either point into the equation. You can use either (-6, 7) or (-9, 8).

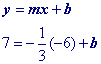
Step 3

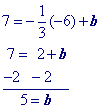
Step 4 the y intercept

Substitute b, 5, into the equation from step 2.

$$ y = \fracx +\red \\ y = \fracx +\red $$

Problem 3

Find the equation of a line through the following the 2 points: (-3, 6) and (15, -6).

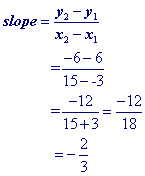
step 1 find slope

Substitute the slope for 'm' in the slope intercept equation.

equation of line

Substitute either point into the equation. You can use either (-3, 6) or (15, -6).

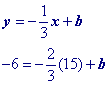
Step 3

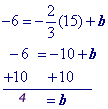
Step 4 the y intercept

Substitute b, -1, into the equation from step 2.

Example 2

Equation from 2 points using Point Slope Form

As explained at the top, point slope form is the easier way to go. Instead of 5 steps, you can find the line's equation in 3 steps, 2 of which are very easy and require nothing more than substitution! In fact, the only calculation, that you're going to make is for the slope.

The main advantage, in this case, is that you do not have to solve for 'b' like you do with slope intercept from.

Find the equation of a line through the points $$(3, 7)$$ and $$(5, 11)$$ .

Substitute the slope for 'm' in the point slope equation.

$$ y - y_1 = m(x - x_1) \\ y - y_1 = \red 2 (x - x_1) $$

Substitute either point as $$ x1, y1 $$ in the equation. You can use either $$(3, 7)$$ or $$ (5, 11) $$ .

Practice Problems - Point Slope

Point Slope is definitely the easier form for what we are doing. It takes 2 steps and 1 of the steps is simply substitution! So, really the only thing you have to do is find the slope and then substitute a point.

Problem 1

Find the equation of a line through the following 2 points: (4, 5) and (8, 7).